
Neither of these are reasonable estimates for the number of blinks a person makes in an hour. 10,000 blinks per hour, which is about three blinks per second. They enable accurate estimations and also enable calculations that go. Consider: 100 blinks per hour, which is about two blinks per minute. In summary, orders of magnitude numbers are typically approximate values. Order of magnitude can be defined as the logarithmic approximation of some value which is related to the reference value contextually understood and represented with base 10 usually. This is an order-of-magnitude estimate, that is, it is an estimate given as a power of ten. Estimation of order of magnitude of a number which is written in scientific notation is calculated by rounding off the power of ten to one greater value if its multiplier value is larger than the value of square root of ten. For example, the estimated value of the number 5000 is ‘4’ because its logarithmic value is 3.698 which is when rounded off to the nearest integer gives ‘4’. Wind plant operators forecast the power they expect to generate by feeding wind speed forecasts from numerical weather models to these power curves 1,2. The great Italian-American nuclear physicist Enrico Fermi (1901-1954) called them back-of the-. The wind turbine power curve relates the speed of wind blowing past a turbine to the power generated by the turbine. On the other hand, the estimates calculated for order of magnitude is the rounding off value to the nearest power of 10. Such calculations are often called order-or-magnitude estimates. We can also check them by calculating the log of these numbers. For example, a subject may be presented with a pure tone of some intensity x presented binaurally, and would be required to estimate the magnitude of the tone on a scale from 1 to 100.
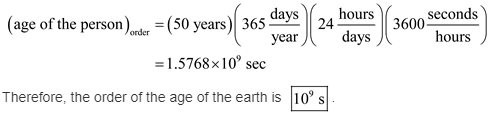
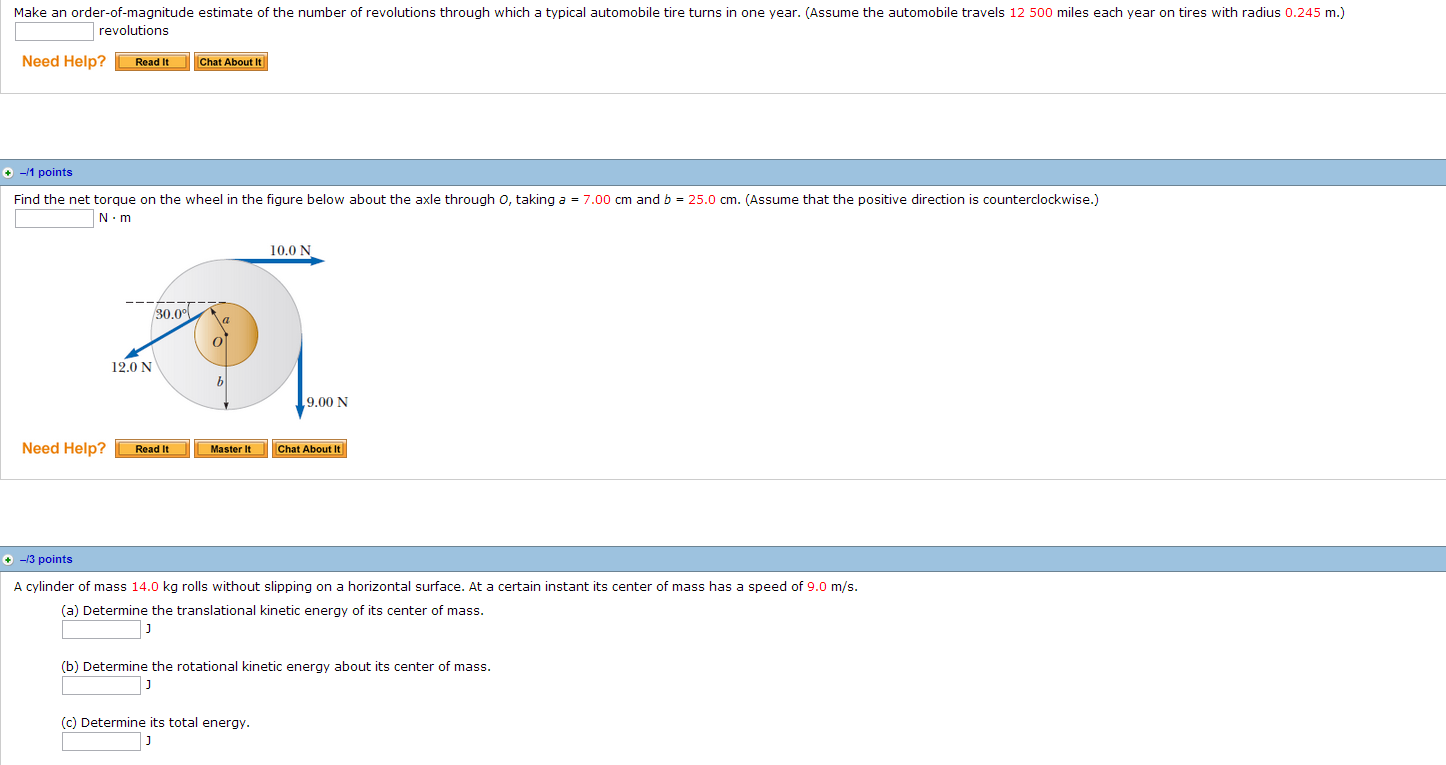
An example of order of magnitude is that if we want to find the order of magnitude of the number 5000, then it will be ‘3’ and for number 700000 it will be ‘5’. In the case of the magnitude estimation method, Stevens (1957) asked his subjects to make direct numerical judgements of the intensities of stimuli. (a) The order of the magnitude of 2800 is 2. Order of magnitude is calculated for making the approximation of the number for simplifying the calculation. Short Answer Estimate the order of magnitude (power of 10) of (a) 2800, (b) 86. In simpler way, any number’s order of magnitude can also be denoted in terms of integer of logarithm which will be calculated by the truncation. Question: Estimate the order of magnitude (power of ten) of: a. Remember, a power of 10 is any number which can be written as 10 multiplied by itself a certain. The order of magnitude of any number is simply the figure of powers on 10 in that number. To get a rough approximation, we find a nearby power of 10.


 0 kommentar(er)
0 kommentar(er)
